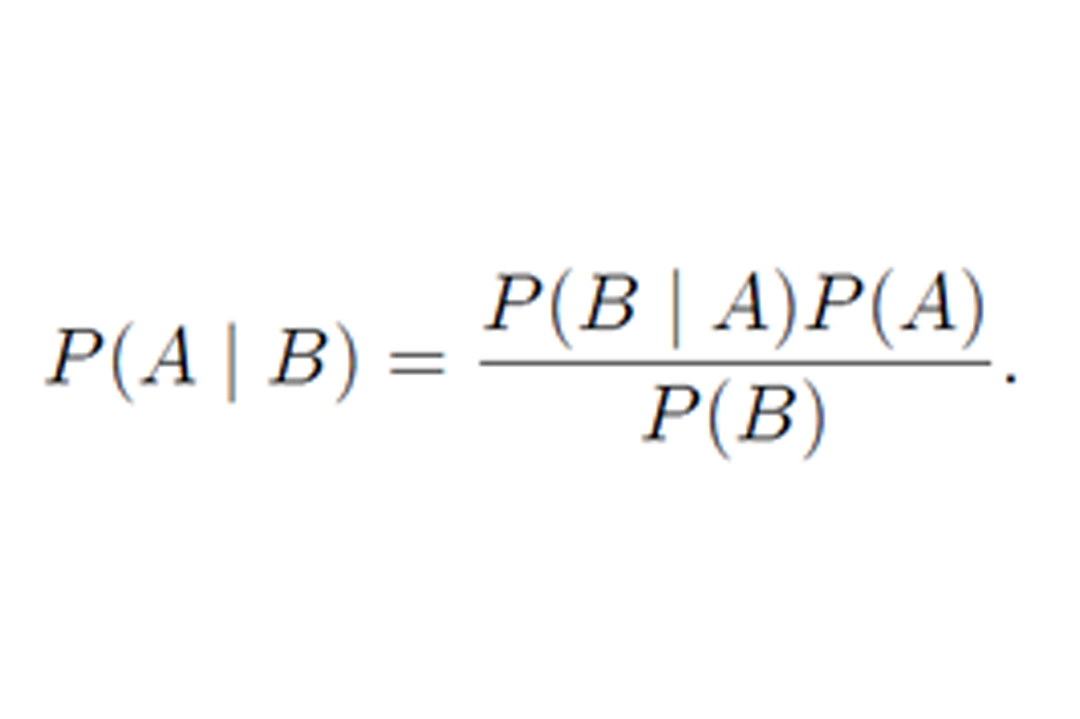Department News, Fall 2014

Message from the Chair
Department Spotlights
Department Announcements
Class Notes
Donor Recognition
Support the Department

 

---

Message from the Chair

 

---

Department Spotlight

Summer Research

Solving the Proton Radius Puzzle

U.S. Physics Olympic Team Comes to GW!

 

---

Department Announcements

Faculty + Research News

QBism! What is it?

by Donald R. Lehman, George Gamow Professor Emeritus of Theoretical Physics

Most knowledgeable people with whom you might discuss quantum mechanics will tell you that it is a marvelously powerful theory that explains the microscopic quantum world with calculational results that are astoundingly accurate no matter what the system: atomic structure, the standard model of particle physics, condensed matter physics, and a lot more. Following its basic principles as to how to perform calculations, no matter whether they are non-relativistic or relativistic, one finds the comparison of the calculational results with experimentally measured results truly stunning. There are no known disagreements. Despite these outcomes, it all seems very formulaic and most of us would like to gain a deeper understanding of quantum theory. When one begins to probe the fundamental aspects of quantum theory a plethora of what seem to be paradoxes arise. The typical questions concern the so-called wave-particle duality, the subtleties of the superposition of wave-function components (think of Schrödinger's cat), wave function collapse, and the idea of two particles being entangled such that a local measurement of the state of one of the particles can have an effect on the state of the other particle located somewhere else in the universe a great distance away. Some physicists may argue that the Copenhagen interpretation of quantum mechanics, originally put forth by Niels Bohr and others, gives us the wherewithal to deal with these situations albeit with limitations on what we can actually know about the microscopic world. On the other hand, especially since about 1990, other physicists have set out to gain a more fundamental understanding of quantum theory or as some would say, its interpretation.

It is worthwhile to list the various attempts at understanding more deeply the foundational aspects of quantum theory. I include in the list the Copenhagen interpretation, dating from the 1920s. The list is:

1. Copenhagen Interpretation – Niels Bohr;
2. Consistent Histories – Robert Griffiths, Roland Omnes, and others;
3. Many-worlds Interpretation – Hugh Everett III;
4. Bohmian Mechanics – David Bohm;
5. Stochastic-evolution interpretation – most recently, Steven Weinberg's attempt at deriving wave function collapse in this framework;
6. Cosmological Interpretation – Anthony Aguirre and Max Tegmark;
7. Quantum Darwinism – Wojciech Zurek;
8. Decoherent Histories – Murray Gell-Mann and James Hartle (This is a variation of Griffiths' consistent histories and in some ways is related to Zurek's work); and
9. QBism – Christopher Fuchs, Carlton Caves, and Rudiger Schack.

The last approach in the list, QBism, is the topic of this short article. QBism is mainly the brainchild of Christopher Fuchs, Carlton Caves, and Rudiger Schack right at the beginning of the 21st century. It is an approach to interpretation that attempts to put the paradoxes referred to above behind us. The approach, known as Quantum Bayesianism shortened to QBism, reinterprets the basic probability amplitude the wave function of quantum theory. Only the meaning of the wave function is changed through a combination of quantum theory with ideas that underpin so-called Bayesian statistics. Within this context, the wave function of quantum theory loses any objective reality that may have been assigned to it in other interpretations of quantum theory. It is important to emphasize that the calculational aspects of quantum theory are unaltered.

Most of us look at probability from the frequentist approach. The frequentist approach looks at the probability of an event through its relative frequency in a series of identically repeated actions (trials). Think of flipping a coin for heads or tails. The number obtained is considered to be objective and almost replicable. Of course, to develop replicable results a large number of trials have to be carried out. Technically, the mathematician will say it actually requires an infinite number of trials to be truly replicable and objective. Therefore, the frequentist approach is not really objective in a philosophically rigorous sense.

The frequentist approach to probability was actually preceded by the subjective approach of an 18th century English clergyman by the name of Thomas Bayes. His ideas were actually developed and used by the French engineer and mathematical physicist Laplace. Central to the Bayesian approach to inference is a theorem (1763). Today, its use for inference seems to be experiencing a strong revival. The theorem is firmly based in sound logical reasoning. Bayes' theorem is basically like an algorithm, where one combines prior knowledge with new evidence in order to calculate the effect of new information on the assessment of probability. The theorem is easily stated in the following manner: If P(A) is the probability of A and P(B) is the probability of B, then the conditional probability of A given B is P(A | B) and the conditional probability of B given A is P(B | A). Bayes' theorem relates these quantities as follows:

Bayes' equation can be interpreted in the following manner: Suppose the initial probability, P(A), is a distribution over some set of propositions given by A in a given hypothesis space. Suppose new evidence is obtained through proposition B. Then, the objective is to update the initial probability distribution owing to the new evidence. Bayes' rule then tells us that the a priori probability, P(A), should be replaced by the conditional probability distribution, P(A | B), which is called the a posteriori probability. Bayes' rule gives us the "algorithm" for calculating the a posteriori probability given P(B | A), which is the inverse probability of the new evidence B. Technically, the denominator is obtained by summing over the original hypotheses as follows:

The posterior probability for A will be higher if the prior probability for A is high or if the quantity P(B | A) is high. Also, if P(B) is low at the starting point, meaning the evidence B is not very strong, then it is unlikely to occur for any of the different hypotheses. This latter situation also leads to a higher posterior probability.

To use this formula, it is obvious that we must know the a priori probability, P(A). Unfortunately, the a priori probability may be "known" by various persons who do not necessarily agree on what the value is, so one must use the best knowledge available to assess P(A). It is through this latter step that subjectivity enters in the Bayes' approach. Note, however, that Bayes' rule can be used iteratively to update the result for P(A | B) given new information about P(A). There are some very nice examples of the application of Bayes' equation in the book by Sharon Bertsch McGrayne entitled The Theory that would not Die – how Bayes' rule cracked the enigma code, hunted down Russian submarines & emerged triumphant from two centuries of controversy (Yale University Press, 2011).

Probabilities within this context are taken to be degrees of belief by an agent about the proposition or propositions involved. Bayes' formula then serves to algorithmically lay out how an agent can learn in a rational manner from measurement results given by B. In quantum theory, the state of the system changes after a measurement is made on the system. This state change can be viewed as an updating process. To describe the measurement of a specific physical quantity, one must know all the possible measurement outcomes and their probabilities. It turns out, thanks to the work of Fuchs and others, that the quantum-mechanical rule for how the state of a system changes upon measurement can be connected to the classical Bayesian approach just discussed. It is done through taking the view that the probabilities associated with a quantum state are subjective degrees of belief. It leads to an equation that is the quantum analog to Eq. (1) above. We will not discuss these details in this short introductory piece but we will attempt to illustrate how this applies in the well-known example of particle entanglement.

The idea of entanglement first came about through a very famous paper authored by Einstein, Podolsky, and Rosen in the mid 1930s. Their work was made more transparent by David Bohm through consideration of a pair of spin-½ particles entangled initially in a state of overall spin-0. It is imagined that this spin-0 state decays into the two spin-½ particles After some period of time, the two particles are considered to be far apart. For purposes of this discussion, we label the particles 1 and 2 to distinguish them. Since these particles were once in contact in a specific state, their spins are highly correlated. If a measurement is made of the spin of particle 1, clearly particle 2 must have the opposite spin (they started in a spin-0 state). Stated simply, even though the particles are far apart, they remain entangled. Once this is accepted, the existence of entanglement in quantum mechanics leads to the question whether measurement on one part of the system can be used to send a message to another distant part without violating the speed-of-light constraint from special relativity. The answer is a resounding "no." There is no instantaneous action at a distance. If Bob measures the spin direction of particle 2, he gets some value but does not know whether some other value was possible. No matter if he repeats the measurement numerous times, he cannot determine what measurements Alice made on particle 1. Particle 1 might have been in a superposition of states without Alice having made any measurements.

Now, let us think about this in the context of the QBism approach. Alice and Bob agree on a particular entangled state as described in the previous paragraph. One particle is located close to Alice and the other close to Bob. Each of them makes a measurement on their nearby particle. In the usual discussion, which is not amplified in the previous paragraph, the outcomes are implicitly assumed to come into existence at the site of each measurement at the moment that measurement is performed (so-called nonlocality effects). From the QBism approach, what is overlooked is that coming into existence of a particular measurement outcome is specific to the agent experiencing the outcome. Alice and Bob both experience an outcome for their own measurement but they can only experience the outcome of the other after they receive the other's communication report (E-mail, telephone call, or the like). Time must elapse for this communication to occur at speeds less than or equal to the speed of light. Experiences of a single agent must be separated by time. Therefore, nonlocality effects do not exist; Alice and Bob both apply quantum mechanics to account for any correlations in two measurement outcomes through their own individual experiences.

Summary
We can succinctly state that QBism takes the subjective or personalist view of probability. This is in contrast to the Copenhagen interpretation where the concept of probability is considered objectively similarly to the frequentist approach. The personal character of probability in QBism emphasizes the personal degrees of belief. QBism is more than just a laboratory measurement Alice can take any set of measurements to determine a set of outcomes. The outcomes guide Alice in updating her probabilities for the next set of measurements she chooses to make. The key point is that the measurement does not reveal a pre-existing state. Alice is acting on the system and producing an outcome, which is a new experience for her. Quantum probabilities are the personal judgment of the agent and the quantum state assignment must also be the personal judgment of the agent assigning the state. The QBism theorists argue that the "collapse" of the wave function is nothing more than the updating of the agent's state assignment on the basis of her experience.

Remark
This article was necessarily short and therefore limited in its content and detail. The interested reader may want to learn more and can do so through the very nice article published in Scientific American by Hans Christian von Baeyer. The article is entitled "Quantum Weirdness? It's All in the Mind." It can be found in the June 2013 Scientific American on page 47. An enthusiastic supporter of the QBism approach is N. David Mermin, who wrote a piece for Physics Today entitled "Quantum Mechanics: Fixing the Shifty Split." His article can be found in Volume 65, No. 7, page 8, published in 2012. The reference to the Caves, Fuchs, and Schack journal article mentioned above is Phys. Rev. A65, 022305 (2002).

Acknowledgement
The author's interest in QBism began after he read Mermin's article in Physics Today. He simultaneously contacted Nozer Singpurwalla, a Bayesian statistician at GW, who is now Professor Emeritus of Statistics and a Chaired Professor at City University of Hong Kong, Ali Eskandarian, who is GW's Dean of the College of Professional Studies and the Dean of the Virginia Science and Technology Campus, and William Parke, Professor Emeritus of Physics. From the initial exchanges of e-mails has come an on-going collaborative study effort among the four of us about the nature of probability within quantum theory and the strengths and weaknesses of the QBism approach. Much remains to be learned and understood at a much deeper level before a final critique of the QBism approach can be constructed. Nevertheless, he is grateful to Nozer, Ali, and Bill for their willingness to think about these issues and serve as critical analysts of his understanding of QBism.

 

---

Alumni Updates/Class Notes

Phil Brinkman, MA ’74: I have worked for the U.S. Department of Transportation since 1973, first for the Federal Highway Administration leading research efforts to develop improved methodologies for evaluating highway safety and, since 1988, with an office now in the Federal Aviation Administration responsible for regulating the commercial space transportation industry to ensure public safety.

Derek Jones, BA ’11: Derek recently completed his MFA in lighting design from Indiana University in May 2014. Since then, he has been a lighting designer based in Washington, D.C., working at venues such as the Kennedy Center and Arena Stage. In December, he will begin a designer position at Vortex Lighting, an architectural lighting design company specializing in hospitality and themed environments based in Los Angeles, Calif. Derek's design portfolio may be seen at www.derekjonesdesignarts.com.

Brandon Minor, BS ’13: I started a PhD program at GW in autonomous robotics. I transferred to CU Boulder this summer, following my advisor Professor Sibley. Two months ago, I left the PhD program entirely to focus my efforts on developing Replica Labs, the company I co-founded. Replica Labs hopes to popularize 3D scanning on mobile phones for a variety of applications. Pursuing the company is a great use of my education and skills.

Silvia Niccolai, PhD ’03: I moved from my native Italy to enroll in a PhD at GW in 1999. I had my PhD graduation in February 2003 in the GW Physics Department. My advisor was the late Professor Berman. The title of my PhD thesis was "Three-body photo-disintegration of 3He measured with CLAS." Since then, I moved to Paris, France, first working as a post-doc (at CEA Saclay and then at the Institute de Physique Nucleaire d'Orsay - IPN). Then, I was hired (October 2006) as a permanent staff researcher at IPN Orsay. I have been working there since, doing research based at Jefferson Lab to study the structure of the nucleon. While doing this, I got married and had twins, a boy and girl who are now almost 5-years-old.

Wallace Yater, BS ’62: I went to G.W from 1956-1962. From 1958-1961, I was a technician in physics and made a lot of the apparatus for the student labs and lecture demonstrations. Over the decades this has gradually dwindled until now nearly none of it can be found any longer. I worked under George Koehl, who was dean of the Columbian College at that time. Professor Bill Parke, who has recently retired, was a close friend. After 1962, I was at the U. S. Navy's David Taylor Model Basin for several years, working in their underwater submarine noise program. For next seven years, I was intermittently at the U. S. Geological Survey's Water Resources Division. In 1972, I moved to a rural area west of Frederick, Md., and focused on mostly architectural custom wood and metal craftsmen's work, most of which still survives. Of these pieces, the Japanese style torii gate in Glen Echo Park is the most easily accessible item of my work in the D.C. area. As you can tell, my efforts didn't do a whole lot to advance our basic understanding of nature, as the work of any self-respecting physicist should, but they might have been of some minor help to a few students and teachers along the way.

 

---

Donor Recognition

The Department of Physics would like to gratefully acknowledge the following generous donors who made a gift to the school from July 1, 2013 – June 30, 2014.

 

---

Support the Department

Gifts to the Physics Department allow us to provide support for faculty and student research and travel, graduate student fellowships, and academic enrichment activities including guest speakers, visiting faculty, and symposia. Each gift, no matter how large or small, makes a positive impact on our educational mission and furthers our standing as one of the nation's preeminent liberal arts colleges at one of the world's preeminent universities.

You can make your gift to the department in a number of ways:

• Give online through our secure donation page.
• Mail your check, made out to the George Washington University and with “Department of Physics” in the memo line, to

The George Washington University
P.O. Box 98131
Washington, DC 20077-9756 

• Call the GW Division of Development and Alumni Relations at 1-800-789-2611 to make a donation by phone.